10 Essential Electrical Laws Every Engineer Should Know

Essential Electrical Laws

Ohm’s Law:

This law defines the relationship between voltage (V), current (I), and resistance (R) in an electrical circuit. Ohm’s Law states that the voltage across a conductor is directly proportional to the current flowing through it, provided the temperature remains constant. This law applies to resistive materials and is fundamental in understanding the relationship between voltage, current, and resistance in electrical circuits.

Formula: V = IR

Where,

V = Voltage (in volts)

I = Current (in amperes)

R = Resistance (in ohms)

Applications: Ohm’s Law is widely used to calculate one of the three quantities (voltage, current, resistance) when the other two are known. It is crucial in designing electrical circuits, particularly in determining the amount of current that flows through components like resistors. It’s fundamental for analyzing and designing electrical circuits.

Kirchhoff’s Laws:

These laws are used to analyze complex electrical circuits. There are two main Kirchhoff’s laws:

Kirchhoff’s Current Law (KCL): Kirchhoff’s Current Law states that the total current entering a junction in a circuit is equal to the total current leaving the junction. In other words, the sum of currents at any node in a circuit is zero. Also known as the junction rule, it’s based on the principle of conservation of charge.

Mathematically: ∑I_in= ∑I_out

Applications: KCL is essential for analyzing parallel circuits and finding current distribution at various nodes. It is used in complex circuit analysis to ensure that current is conserved.

Kirchhoff’s Voltage Law (KVL): Kirchhoff’s Voltage Law states that the sum of the electrical potential differences (voltage) around any closed loop or mesh in a circuit is zero. This means that the total voltage supplied is used up by resistive elements and other components in the loop.

Mathematically: ∑ V=0 around any closed loop.

Applications: KVL helps in analyzing the distribution of voltages in a series circuit and is fundamental in mesh analysis, especially in circuits with multiple components like resistors, capacitors, and inductors.

Coulomb’s Law:

Coulomb’s Law describes the force between two charges. It states that the force between two point charges is directly proportional to the product of their charges and inversely proportional to the square of the distance between them. The force is attractive if the charges are opposite, and repulsive if the charges are of the same sign.

Formula:

F = k⋅q₁⋅q₂ / r²

Here,

F = Force between two charges (in newton’s)
q₁, q₂ = The amounts of the two charges (in coulombs)
r = Distance between the charges (in meters)
k = Coulomb’s constant (8.99×10⁹ N m²/C²)

Applications: Coulomb’s Law is fundamental in understanding electric fields and the behavior of charges in an electric field. It is applied in electrostatics, including in capacitors and electrostatic forces.

Faraday’s Law of Electromagnetic Induction:

Faraday’s Law states that a change in magnetic flux through a loop induces an electromotive force (EMF) in the loop. The induced EMF is proportional to the rate of change of the magnetic flux through the loop. The negative sign in the formula indicates the direction of the induced EMF opposes the change in flux (Lenz’s Law).

Formula: ϵ =−dΦ / dt

ε = Induced EMF (in volts)
Φ = Magnetic flux (in Weber)
t = Time (in seconds)

Applications: Faraday’s Law is used in the operation of electrical generators, transformers, and inductors. It is fundamental in the design of devices that convert mechanical energy to electrical energy.

5. Lenz’s Law:

Lenz’s Law is a consequence of Faraday’s Law of Induction. It states that the direction of the induced current (or EMF) will always oppose the change in magnetic flux that produced it. This law ensures the conservation of energy in electromagnetic processes.

Applications: Lenz’s Law is important in understanding the behavior of inductive elements in circuits, such as inductors and transformers. It is used to explain why motors and generators work in a way that resists changes to the magnetic field.

Gauss’s Law:

Description:
- This law relates the distribution of electric charge to the resulting electric field.
Significance:
- It’s a powerful tool for calculating electric fields in various situations.

7. Capacitance Law:

The law of capacitance states that the capacitance of a capacitor is the ratio of the charge stored on one of the plates to the potential difference between the plates. Capacitance is a measure of a capacitor’s ability to store charge.

Formula: C=Q / V

C = Capacitance (in farads)
Q = Charge stored on the plates (in coulombs)
V = Voltage across the plates (in volts)

Applications: This law is essential in the design and analysis of capacitors, which are used in many electronic circuits to store energy, filter signals, and smooth out voltage fluctuations.

What is Biot savart law?

The Biot-Savart Law is a fundamental principle in electromagnetism that describes the magnetic field generated by a moving electric charge or a current-carrying conductor. It provides a mathematical relationship for calculating the magnetic field at any point in space due to a small segment of current.

In its most general form, the Biot-Savart Law states that the magnetic field B at a point in space is proportional to the current III in a wire segment, the length of that segment, and the angle between the segment and the point in question. The law is often written as:

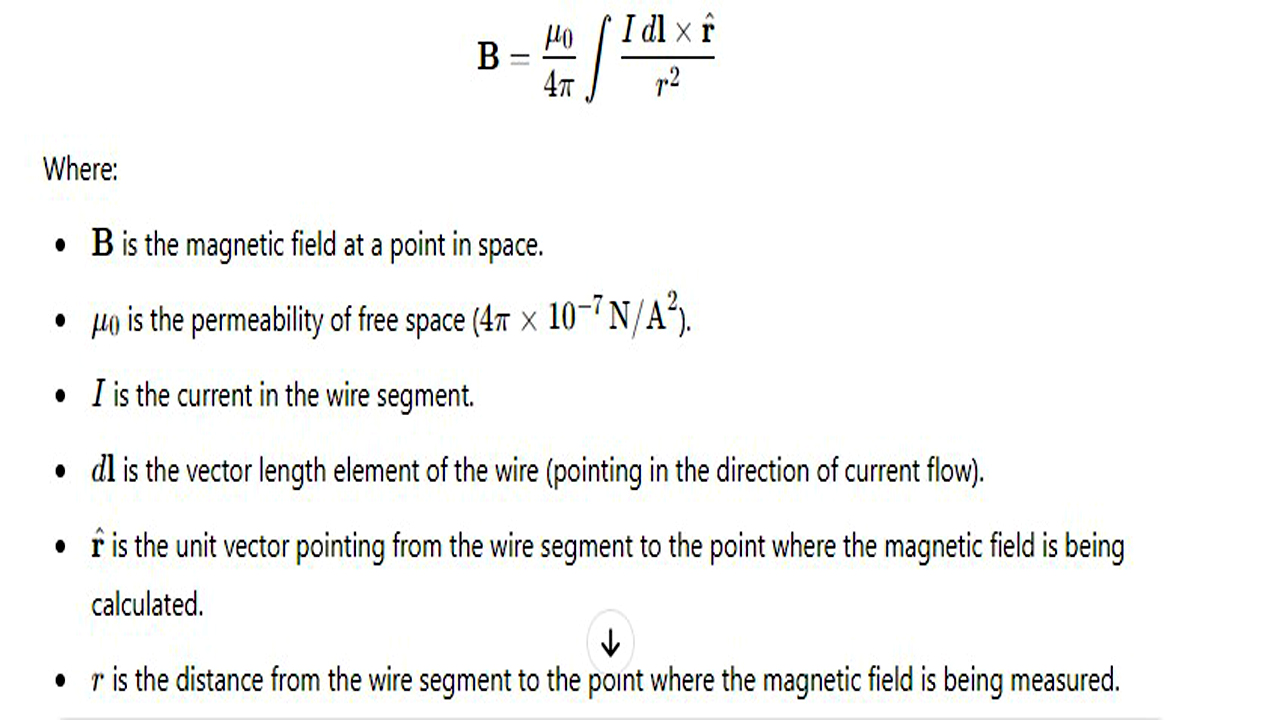
Purpose:

The Biot-Savart law provides a way to calculate the contribution of a small segment of a current-carrying wire to the overall magnetic field. By summing up the contributions from all such segments, we can find the total magnetic field.

It allows us to calculate the magnetic field produced by a current-carrying conductor.
It helps determine the magnitude and direction of the magnetic field at a specific point in space due to an electric current.

Key Points:

The magnetic field produced by a current is a result of the motion of charged particles (electrons).
The direction of the magnetic field is given by the right-hand rule: if the thumb of the right hand points in the direction of the current, the curled fingers show the direction of the magnetic field.
The magnitude of the magnetic field decreases with the square of the distance from the current-carrying segment.

The Biot-Savart Law is particularly useful for calculating magnetic fields due to arbitrary current distributions, such as straight wires, loops, and solenoids.

9. Power Law:

The Power Law states that the electrical power consumed by an electrical component or circuit is the product of the current flowing through the component and the voltage across it. Power is measured in watts (W).

Formula:

P= VI

P = Power (in watts)
I = Current (in amperes)
V = Voltage (in volts)

Applications: The Power Law is used in calculating the energy consumption of electrical devices and in understanding the efficiency of electrical systems. It’s also essential in the design of power transmission and distribution systems.

10. Thevenin’s and Norton’s Theorems:

These are simplifying tools used in circuit analysis.

a) Thevenin’s Theorem:

It states that any complex linear circuit can be replaced by an equivalent circuit consisting of a single voltage source (Thevenin voltage) in series with a resistance (Thevenin resistance) when viewed from the terminals.

b) Norton’s Theorem:

Similar to Thevenin’s theorem, Norton’s theorem states that any linear circuit can be replaced by an equivalent circuit consisting of a current source (Norton current) in parallel with a resistance (Norton resistance).

Applications: These theorems simplify the analysis of complex circuits by reducing them to simpler equivalent circuits, making it easier to calculate current and voltage values at different parts of the circuit.

10 Essential Electrical Laws Every Engineer Should Know

Essential Electrical Laws

Ohm’s Law:

Kirchhoff’s Laws:

Coulomb’s Law: